“I got another question, Moire.”
“Of course you do, Mr Feder.”
“When someone’s far away they look smaller, right, and when someone’s standing near a black hole they look smaller, too. How’s the black hole any different?”
“The short answer is, perspective depends on the distance between the object and you, but space compression depends on the distance between the object and the space-distorting mass. The long answer’s more interesting.”
“And you’re gonna tell it to me, right?”
“Of course. I never let a teachable moment pass by. Remember the August eclipse?”
“Do I? I was stuck in that traffic for hours.”
“How’s it work then?”
“The eclipse? The Moon gets in front of the Sun and puts us in its shadow. ‘S weird how they’re both the same size so we can see the Sun’s corundum and protuberances.”
“Corona and prominences. Is the Moon really the same size as the Sun?”
“Naw, I know better than that. Like they said on TV, the Moon’s about ¼ the Earth’s width and the Sun’s about 100 times bigger than us. It’s just they look the same size when they meet up.”
“So the diameter ratio is about 400-to-1. Off the top of your head, do you know their distances from us?”
“Millions of miles, right?”
“Not so much, at least for the Moon. It’s a bit less than ¼ of a million miles away. The Sun’s a bit less than 100 million miles away.”
“I see where you’re going here — the distances are the same 400-to-1 ratio.”
“Bingo. The Moon’s actual size is 400 times smaller than the Sun’s, but perspective reduces the Sun’s visual size by the same ratio and we can enjoy eclipses. Let’s try another one. To keep the arithmetic simple I’m going to call that almost-100-million-mile distance an Astronomical Unit. OK?”
“No problemo.”
“Jupiter’s diameter is about 10% of the Sun’s, and Jupiter is about 5 AUs away from the Sun. How far behind Jupiter would we have to stand to get a nice eclipse?”
“Oh, you’re making me work, too, huh? OK, I gotta shrink the Sun by a factor of 10 to match the size of Jupiter so we gotta pull back from Jupiter by the same factor of 10 times its distance from the Sun … fifty of those AUs.”
“You got it. And by the way, that 55 AU total is just outside the farthest point of Pluto’s orbit. It took the New Horizons spacecraft nine years to get there. Anyhow, perspective’s all about simple ratios and proportions, straight lines all the way. So … on to space compression, which isn’t.”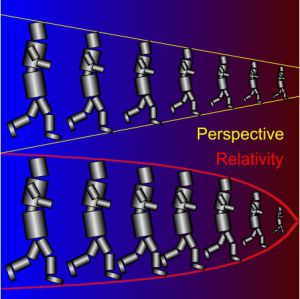
“We’re not going to do calculus, are we?”
“Nope, just some algebra. And I’m going to simplify things just a little by saying that our black hole doesn’t spin and has no charge, and the object we’re watching, say a survey robot, is small relative to the black hole’s diameter. Of course, it’s also completely outside the event horizon or else we couldn’t see it. With me?”
“I suppose.”
“OK, given all that, suppose the robot’s as-built height is h and it’s a distance r away from the geometric center of an event horizon’s sphere. The radius of the sphere is rs. Looking down from our spaceship we’d see the robot’s height h’ as something smaller than h by a factor that depends on r. There’s a couple of different ways to write the factor. The formula I like best is h’=h√[(r-rs)/r].”
“Hey, (r-rs) inside the brackets is the robot’s distance to the event horizon.”
“Well-spotted, Mr Feder. We’re dividing that length by the distance from the event horizon’s geometric center. If the robot’s far away so that r>>rs, then (r-rs)/r is essentially 1.0 and h’=h. We and the robot would agree on its height. But as the robot closes in, that ratio really gets small. In our frame the robot’s shrinking even though in its frame its height doesn’t change.”
“We’d see it getting smaller because of perspective, too, right?”
“Sure, but toward the end relativity shrinks the robot even faster than perspective does.”
“Poor robot.”
~~ Rich Olcott
- Thanks to Carol, who inspired this post by asking Mr Feder’s question but in more precise form.





